Aristotelous

Research

 

My research interests are focused on the development, theoretical analysis and implementation of efficient algorithms in view of their application to the simulation of problems of practical interest. My specialty is on Discontinuous Galerkin Finite Element Methods (DG-FE) methods for the numerical solution of partial differential equation (PDE) problems and applications. Specifically, I am interested in the development and analysis of high order numerical schemes that accurately and efficiently resolve the solution to non linear equations like Cahn-Hilliard type equations, arising in models describing biological growth e.g. cancerous tumors and phase separation. Adaptive mesh refinement, (theoretical) error analysis and energy stability via convex splitting are also being addressed in my work. For the solution of the resulting algebraic (nonlinear) systems efficient solvers based on multigrid are designed. Recently the development and application of machine learning methods for the numerical solution of partial differential equations is an interesting aspect of my work.

The development of hybrid discrete cellular automata systems (HD-CA) by coupling linear and non-linear reaction diffusion partial differential equations with discrete (stochastic) cellular automata systems is also a part of my work. I utilize hybrid discrete systems to derive constitutive relationships and study phenomena in biology specifically biofilm, cancer, tissue engineering, virus dynamics modeling, and evolutionary game theory.

I am looking for motivated students with love for mathematics that are interested in doing research. Ask me for more details!!

 

External Funding

  • As PI: 2017-2020,  NSF-DMS 1720226, Awarded $100,115.00, Title: “Computational Methods for Heterogeneous Soft Living Materials” (Link)

Current Research Projects

  • Discontinuous Galerkin Finite Element methods for the numerical solution of various Cahn-Hilliard type equations
    Work involves development, convergence analysis (error estimates) and implementation of numerical schemes in primitive variable and mixed formulations, utilizing adaptive mesh refinement and fast linear and nonlinear system solvers.
  • With Professor’s Vasileios Maroulas group, University of Tennessee Mathematics: Working on machine learning for PDEs.
  • With Professor’s Gregory Forest group, UNC Mathematics: We are interested in modeling the SARS-CoV-2 progression in the respiratory track.

Publications

Machine Learning for PDEs

 

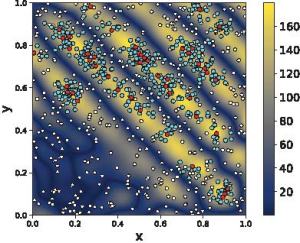
  • Aristotelous, A. C., Mitchell, E. C., Maroulas, V. (2023) ADLGM: An efficient adaptive sampling deep learning Galerkin method, Journal of Computational Physics, 111944. (Link)

  • Aristotelous, A. C. & Papanicolaou, N. C. (2023) On the Transferability of the Deep Galerkin Method for Solving Partial Differential Equations.
    American Institute of Physics (AIP) Conference Proceedings, 2953, 090001, (Link).

 

Hybrid Discrete Systems

 

 

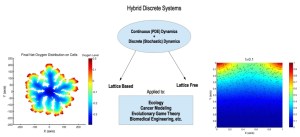
  • Aristotelous, A. C., Chen, A., Forest, M. G. (2022) A hybrid discrete-continuum model of immune responses to SARS-CoV-2 infection in the lung alveolar region, with a focus on interferon induced innate response, Journal of Theoretical Biology, 111293. (Link)

  • Aristotelous, A. C. (2022) Biofilm neutrophils interactions under hypoxia: A mathematical modeling study. Mathematical Biosciences, 352, 108893. (Link)

  • Aristotelous, A. C., Grabovsky, Y. & Klapper, I. (2018) Heterogeneity Formation Within Biofilm Systems. European Journal of Applied Mathematics, 1-15. (Link)
  • Aristotelous, A. C., Klapper, I., Grabovsky, Y., Pabst, B., Pitts, B. and Stewart, P. S. (2015) Diffusive Transport Through Host-Biofilm Systems. Phys. Rev. E , 92-2, 022703. (Link)
  • Aristotelous, A. C. and Durrett, R. (2014) Fingering in Stochastic Growth Models. Experimental Mathematics, 23-4, 465–474. (Link)
  • Aristotelous, A. C. and Haider, M. A. (2014) Evaluation of Diffusive Transport and Cellular Uptake of Nutrients in Tissue Engineered Constructs using a Hybrid Discrete Model. Processes: Special Issue “Design of bioreactor systems for tissue engineering”, 2-2, 333–344. (Link)
  • Aristotelous, A. C. and Durrett, R. (2014) Chemical Evolutionary Games. Theoretical Population Biology, 93, 1–13. (Link)
  • Aristotelous, A. C. and Haider, M. A. (2014) Use of Hybrid Discrete Cellular Models for Identification of Macroscopic Nutrient Loss in Reaction-Diffusion Models of Tissues. Int. J. Numer. Meth. Biomed. Engng., 30-8,767–780. (Link)

 

Numerical Analysis

 

 

  • Aristotelous, A. C. (2023) Energy Stable Symmetric Interior Penalty Discontinuous Galerkin Finite Element for a Growth Cahn-Hilliard Equation.
    American Institute of Physics (AIP) Conference Proceedings, 2953, 080001 (Link).

  • Aristotelous, A. C., Karakashian, O. A. and Wise, S. M. (2015) Adaptive, Second-Order in Time, Primitive-Variable Discontinuous Galerkin Schemes for a Cahn-Hilliard Equation with a Mass Source. IMA J. Numer. Anal., 35-3, 1167–1198. (Link)

  • Aristotelous, A. C., Karakashian, O. A. and Wise, S. M. (2013) A Mixed Discontinuous Galerkin, Convex Splitting Scheme for a Modified Cahn-Hilliard Equation and an Efficient Nonlinear Multigrid Solver. DCDS-B, 18-9, 2211–2238. (Link)

 

Computational Models

  • A. C. Aristotelous & N. C. Papanicolaou (2017) A Numerical Study of Biofilm Growth in a Microgravity Environment. American Institute of Physics (AIP) Conference Proceedings, 1895, 120001. (Link)

 

Computational Fluid Dynamics

  • Aristotelous, A. C. & Papanicolaou, N. C. (2016) A Discontinuous Galerkin Method for Unsteady Two-dimensional Convective Flows. American Institute of Physics (AIP) Conference Proceedings, 1773, 110002. (Link)
  • Papanicolaou, N. C. and Aristotelous, A. C. (2015) High-Order Discontinuous Galerkin Methods for Coupled Thermoconvective Flows under Gravity Modulation. American Institute of Physics (AIP) Conference Proceedings 1684., 090010. (Link)

 

Review Papers

  • Aristotelous, A. C., Crawford, J. M., Edwards, G. S., Kiehart, D. P. & Venakides, S. (2018) Mathematical models of dorsal closure. Progress in Biophysics and Molecular Biology,  137, 111–131 (Link)
  • Daniel P. Kiehart, Janice M. Crawford, Andreas C. Aristotelous, Stephanos Venakides & Glenn S. Edwards (2017) Cell Sheet Morphogenesis: Dorsal Closure in Drosophila Melanogaster as a Model System. Annual Review of Cellular and Developmental Biology, 33-1, 169–202. (Link)

 

PhD Dissertation

  • Aristotelous, A. C. (2011) Adaptive Discontinuous Galerkin Finite Element Methods for a Diffuse Interface Model of Biological Growth. PhD Thesis, The University of Tennessee, U.S.A. (Link)